Plot of the six trigonometric functions, the unit circle, and a line for the angle = radians The points labelled 1, Sec(θ), Csc(θ) represent the length of the line segment from the origin to that point Sin(θ), Tan(θ), and 1 are the heights to the line starting from the axis, while Cos(θ), 1, and Cot(θ) are lengths along the axis starting from the originFree trigonometric identities list trigonometric identities by request stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy Learn more Accept Solutions Graphing Practice;Identities expressing trig functions in terms of their supplements Sum, difference, and double angle formulas for tangent The half angle formulas The ones for sine and cosine take the positive or negative square root depending on the quadrant of the angle θ/2 For example, if θ/2 is an acute angle, then the positive root would be used Truly obscure identities These are just here for
What Is The Formula Of Tan2x Quora
Tan^2x trig identity
Tan^2x trig identity-Now use the identity to replace sin 2 x and write the new integral Now this new integral is a sum of two integrals, the last of which can be evaluated easily using the substitution u = cos(x), like this The first integral is easy, it's just cos(x) The second is easy because of the substitution Now we just back substitute cos(x) for u to get the solution (don't forget the constant) WeAs there is no way to immediately integrate tan^2 (x) using well known trigonometric integrals and derivatives, it seems like a good idea would be writing tan^2 (x) as sec^2 (x) 1 Now, we can recognise sec^2 (x) as the derivative of tan (x) (you can prove this using the quotient rule and the identity sin^2 (x) cos^2 (x) = 1), while we get




How To Use Double Angle Identities Studypug
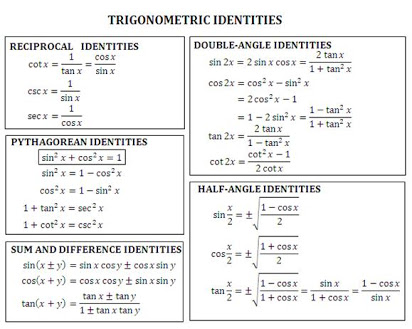
Tan(xy)= tan(x)tan(y) 1tan(x)tan(y) DIFFERENCE IDENTITIES sin(xy)=sin(x)cos(y)cos(x)sin(y) cos(xy) = cos(x)cos(y)sin(x)sin(y) tan(xy)= tan(x)tan(y) 1tan(x)tan(y) LAW OF SINES sin(A) a = sin(B) b = sin c DOUBLEANGLE IDENTITIES sin(2x)=2sin(x)cos(x) cos(2x) = cos2(x)sin2(x) = 2cos2(x)1 =12sin2(x) tan(2x)= 2tan(x) 1 2tan (x) HALFANGLETan(x y) = (tan x tan y) / (1 tan x tan y) sin(2x) = 2 sin x cos x cos(2x) = cos 2 (x) sin 2 (x) = 2 cos 2 (x) 1 = 1 2 sin 2 (x) tan(2x) = 2 tan(x) / (1Therefore in mathematics as well as in physics, such formulae are useful for deriving many important identities The trigonometric formulas like Sin2x, Cos 2x, Tan 2x are popular as double angle formulae, because they have double angles in their trigonometric functions For solving many problems we may use these widely The Sin 2x formula is \(Sin 2x = 2 sin x cos x\) Where x
In this video I go over the proof of the trigonometry identity tan^2(x) 1 = sec^2(x) The proof of this identity is very simple and like many other trig id Trig identity $1\tan x \tan 2x = \sec 2x$ 13 Which trigonometric identities involve trigonometric functions?Trig identities tan^2Trigonometric Identities Pythagoras's theorem sin2 cos2 = 1 (1) 1 cot2 = cosec2 (2) tan2 1 = sec2 (3) Note that (2) = (1)=sin 2 and (3) = (1)=cos CompoundThe half‐angle identity for tangent can be written in three different forms In the first form, the sign is determined by the quadrant in which the angle α/2 is located Example 5 Verify the identity Example 6 Verify
Here we will prove the problems on trigonometric identities As you know that the identity consists of two sides in equation, named Left Hand Side (abbreviated as LHS) and Right Hand Side (abbreviated as RHS)To prove the identity, sometimes we need to apply more fundamental identities, eg $\sin^2 x \cos^2 x = 1$ and use logical steps in order to lead oneTrig Identities Identities involving trig functions are listed below Pythagorean Identities sin 2 θ tan (x π) = tan x cot (x π) = cot x tan (x 180°) = tan x cot (x 180°) = cot x Sum/Difference Identities Double Angle Identities Half Angle Identities or or or or Product to Sum Identities Sum to Product Identities See also Sine, cosine, tangent, cosecantTrig identity $1\tan x \tan 2x = \sec 2x$ Ask Question Asked 10 years, 3 months ago Active 6 years, 1 month ago Viewed 6k times 3 0 $\begingroup$ I need to prove that $$1\tan x \tan 2x = \sec 2x$$ I started this by making sec 1/cos and using the double angle identity for that and it didn't work at all in any way ever Not sure why I can't do that, but something was wrong



1



Trig Identities Table Of Trigonometric Identities
Proving a trigonometric identity refers to showing that the identity is always true, no matter what value of x x x or θ \theta θ is used Because it has to hold true for all values of x x x, we cannot simply substitute in a few values of x x x to "show" that they are equal It is possible that both sides are equal at several values (namely when we solve the equation), and we might falsely2tan2 x = sec2 x for values of x in the interval 0 ≤ x < 2π We try to relate the given equation to one of our three identities We can use the identity sec2 x = 1tan2 x to rewrite the equation solely in terms of tanx 2tan2 x = sec2 x 2tan 2x = 1tan x from which tan2 x = 1 Taking the square root then gives tanx = 1 or − 1Definitions Trigonometric functions specify the relationships between side lengths and interior angles of a right triangle For example, the sine of angle θ is defined as being the length of the opposite side divided by the length of the hypotenuse The six trigonometric functions are defined for every real number, except, for some of them




Trigonometric Identities Simplify Expressions Video Lessons Examples And Solutions




Trig Identities Turn The Wheel
Account Details Login Options Account ManagementAccount Details Login Options Account Management True Start with the well known pythagorean identity sin^2x cos^2x = 1 This is readily derived directly from the definition of the basic trigonometric functions sin and cos and Pythagoras's Theorem Divide both side by cos^2x and we get sin^2x/cos^2x cos^2x/cos^2x = 1/cos^2x tan^2x 1 = sec^2x tan^2x = sec^2x 1 Confirming that the result is an identity




Solved 2 Prove The Following Trig Identities A Prove Tan Chegg Com




Integrate Sec 2x Method 2
Free trigonometric identity calculator verify trigonometric identities stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy Learn more Accept Solutions Graphing Practice;Trig Identities STUDY Flashcards Learn Write Spell Test PLAY Match Gravity Created by connorbassett1 Terms in this set (32) Pythagorean Identity sin^2xcos^2x=1 Pythagorean Identity 1tan^2x=sec^2x Pythagorean Identity 1cot^2x=csc^2x Quotient Identity of Tangent tanθ = sinθ/cosθ Quotient Identity of Cotangent cotθ = cosθ/sinθ Reciprocal$\sec^2{x}\tan^2{x} \,=\, 1$ $\sec^2{A}\tan^2{A} \,=\, 1$ Remember, the angle of a right triangle can be represented by any symbol but the relationship between secant and tan functions must be written in that symbol Proof Learn how to prove the Pythagorean identity of secant and tan functions in mathematical form by geometrical method




Tan 2x Csc 2x Tan 2x 1 Problem Solving Solving Identity



Rlemke01 Math Tufts Edu
Power reducing Identity tan^2 x 1 cos (2x) / 1 cos (2x) half angle formula for sine sin (x/2) = / square root of ( 1cosx ) / 2 half angle formula for cosine cos (x/2) = / square root of ( 1 cosx ) / 2 half angle formula for tangent tan (x/2) = ( 1cosx ) / (sinx)This is probably the most important trig identity Identities expressing trig functions in terms of their complements There's not muchPDF Trigonometric identities are mathematical equations which are made up of functions These identities are true for any value of the variable put There are many identities which are derived by the basic functions, ie, sin, cos, tan, etc The most basic identity is the Pythagorean Identity, which is derived from the Pythagoras Theorem Trigonometric Identities and Formulas by James Lowman Trigonometric identities are equations that are true for every value of the variable, or variables, that involve trigonometric functions




Trig Identity Sec2x Minus Tan2x T10 Youtube
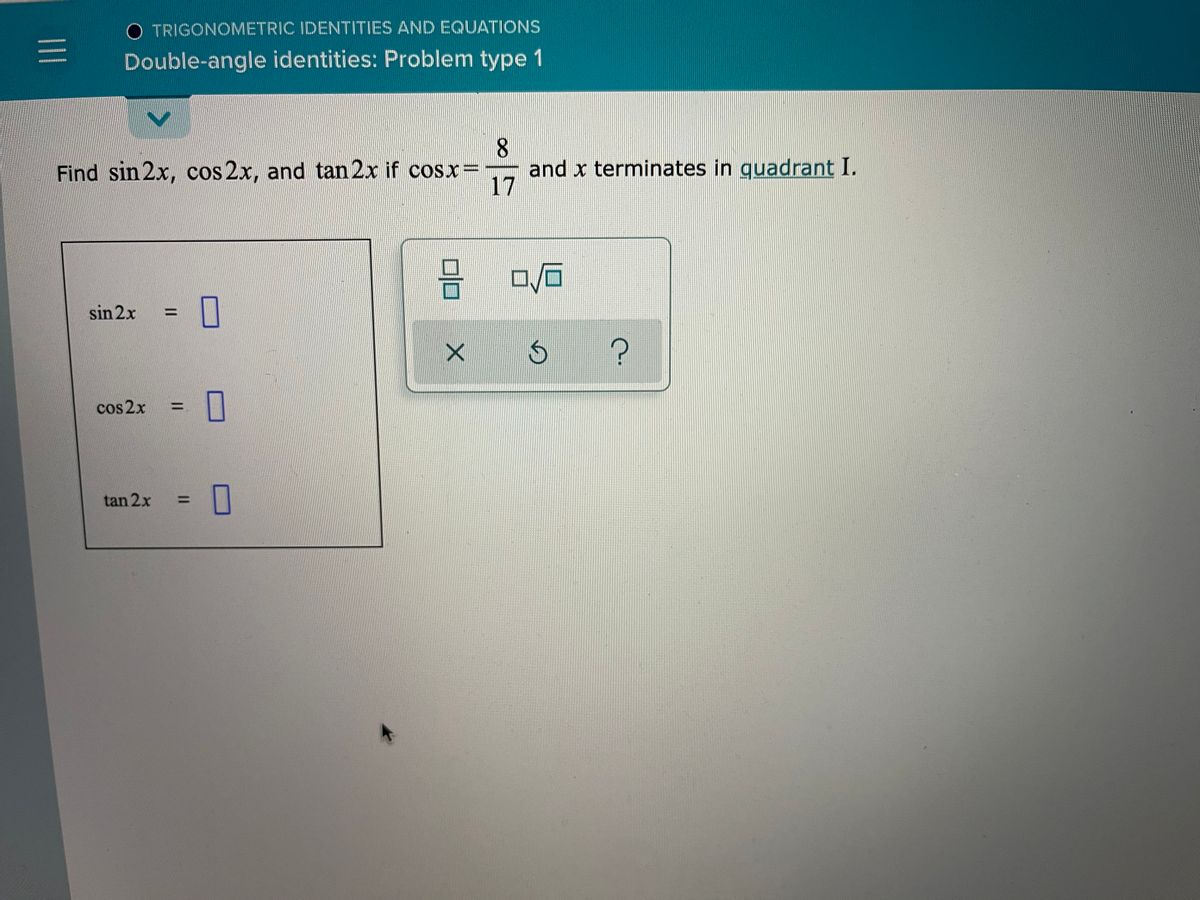



Answered O Trigonometric Identities And Bartleby



0 件のコメント:
コメントを投稿