According to Pascal's Triangle, the coefficients for (xy)^3 are 1, 3, 3, 1 This means that the expansion of (xy)^3 will be R^2 at SCCFactor x^3y^3 x3 − y3 x 3 y 3 Since both terms are perfect cubes, factor using the difference of cubes formula, a3 −b3 = (a−b)(a2 abb2) a 3 b 3 = ( a b) ( a 2 a b b 2) where a = x a = x and b = y b = y (x−y)(x2 xyy2) ( x y) ( x 2 x y y 2) Definition binomial A binomial is an algebraic expression containing 2 terms For example, (x y) is a binomial We sometimes need to expand binomials as follows (a b) 0 = 1(a b) 1 = a b(a b) 2 = a 2 2ab b 2(a b) 3 = a 3 3a 2 b 3ab 2 b 3(a b) 4 = a 4 4a 3 b 6a 2 b 2 4ab 3 b 4(a b) 5 = a 5 5a 4 b 10a 3 b 2 10a 2 b 3 5ab 4 b 5Clearly, doing

Binomial Theorem By Calum Blair Issuu
(x+y-1)^3 expansion
(x+y-1)^3 expansion-NOTE Shapeoko 3 Expansion Packs will only be available through Nov 21 Convert your standard Shapeoko 3 to a Shapeoko XL or Shapeoko XXL Cutting Area (XL) 33"(X), 17"(Y), 3"(Z) Cutting Area (XXL) 33"(X) x 33"(Y) x 3"(Z) The kit includes everything you need to convert your standard machine to a larger versionX^3 y^3 z^3 3x^2y 3xy^2 3x^2z 3z^2x 3y^2z 3z^2y 6xyz Lennox Obuong Algebra Student Email obuong3@aolcom




Expand X Y 3 Solved
Free math lessons and math homework help from basic math to algebra, geometry and beyond Students, teachers, parents, and everyone can find solutions to their math problems instantlyX y is a binomial in which x and y are two terms In mathematics, the cube of sum of two terms is expressed as the cube of binomial x y It is read as x plus y whole cube It is mainly used in mathematics as a formula for expanding cube of sum of any two terms in their terms ( x y) 3 = x 3 y 3 3 x 2 y 3 x y 2 Find the coefficient of the term x^6y^3 in the expansion of (x2y)^9 asked in Mathematics by Abdulazeez John Bami (122 points) binomial theorem;
We know that General term of expansion (a b)n is Tr1 = nCr an–r br For (x 2y)9, Putting n = 9 , a = x , b = 2y Tr 1 = 9Cr (x)9 – r (2y)r = 9Cr (x)9 – r (y)r (2)r We need to find coefficient of x6 y3 Comparing yr = y3 r = 3 Putting r = 3 in (1) T31 = 9C3 x9 – 3 y3Problems on Binomial Theorem Question 1 If the third term in the binomial expansion of equals 2560, find x Solution ⇒ (log 2x) 2 = 4 ⇒ log 2x = 2 or 2 ⇒ x = 4 or 1/4 Question 2 Find the positive value of λ for which the coefficient of x2 in the expression x2√ x1 Inform you about time table of exam 2 Inform you about new question papers 3 New video tutorials information
Expand (xy)^3 (x y)3 ( x y) 3 Use the Binomial Theorem x3 3x2y3xy2 y3 x 3 3 x 2 y 3 x y 2 y 3 Row 10 1 10 45 1 210 1x^10 10x^9y^1 45x^8y^2 1x^7y^3 If you do it by combinations, you want 10nCr7 = 1 (the 10 comes from row 10, determined by the sum of the two exponents, the 7 comes from the exponent of the xWhy create a profile on Shaalaacom?




16 Pokemon Xy Steam Siege Expansion Set Base 3 Hoppip




Expand And Simplify Binomial Squares 2x 3y 2 Youtube
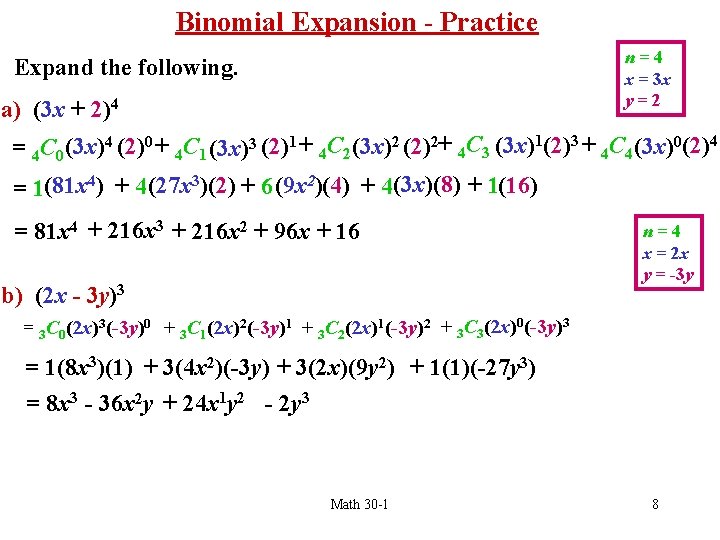
Determinea) the first 3 terms in the binomial expansion of ( x y − 4 x) 5b) the first 2 terms in the binomial expansion of ( 2 a 2 b 3) 9c) the last 3 terms in the binomial expansion of ( 3 y 2 − x 2) 7d) the first 3 terms in the binomial expansion of ( x y − y x) 10e) the last 4 terms in the binomial expansion of ( m 3 n 1 2 n 2) 6Question What Is The Coefficient Of X^3 Y^4 In The Expansion Of (2x Y 5)^8?Binomial Expansions Binomial Expansions Notice that (x y) 0 = 1 (x y) 2 = x 2 2xy y 2 (x y) 3 = x 3 3x 3 y 3xy 2 y 3 (x y) 4 = x 4 4x 3 y 6x 2 y 2 4xy 3 y 4 Notice that the powers are descending in x and ascending in yAlthough FOILing is one way to solve these problems, there is a much easier way




Pokemon Tcg Xy Breakpoint Expansion Revealed Gotgame




Find The Coefficient Of X 6y 3in The Expansion Of X 2y 9
\(xy)^3 = \binom{3}{0} x^3 \binom{3}{1} x^2 y \binom{3}{2} xy^2 \binom{3}{3} y^3\nonumber\ Finally, evaluate the binomial coefficients and simplify the result \(xy)^3 = x^3 3x^2y 3xy^2 y^3\nonumber\ In a similar way, we also find \((xy)^3 = x^3 3x^2y 3xy^2 y^3\) Note the similarity between the two expansionsUtilize the Binomial Expansion Calculator and enter your input term in the input field ie, $(11xy)^3$ & press the calculate button to get the result ie, $1331x^3 363x^2y 33xy^2 y^3$ along with a detailed solution in a fraction of seconds Ex (x1)^2 (or) (x7)^7 (or) (x3)^4 What is the coefficient of x^2y^3 in the expansion of (2xy)^5 The Lualailua Hills Quadrangle of the East Maui (Haleakala)
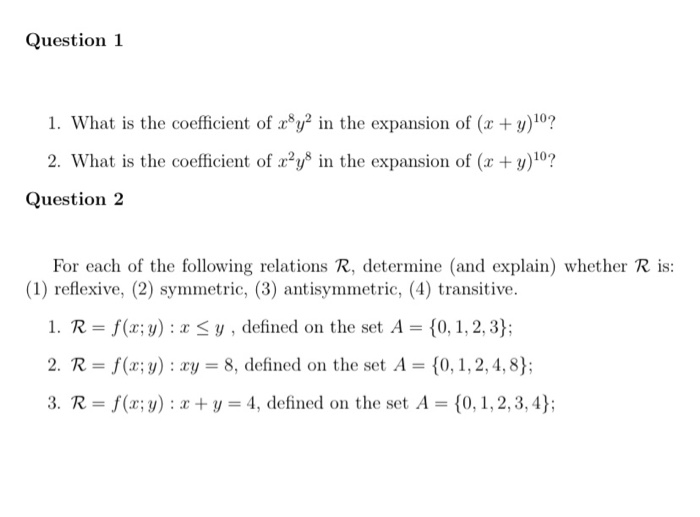



Question 1 1 What Is The Coefficient Of Roy2 In The Chegg Com




How To Expand Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube
Binomial Theorem Formula When the number of terms is odd, then there is a middle term in the expansion in which the exponents of a and b are the same Only in (a) and (d), there are terms in which the exponents of the factors are the sameMentally examine the expansion of math(xyz)^3/math and realize that each term of the expansion must be of degree three and that because mathxyz/math is cyclic all possible such terms must appear Those types of terms can be representedFind the coefficent of`x^(6)y^(3)` in the expansion of `(x2y)^(9)` Step by step solution by experts to help you in doubt clearance & scoring excellent marks in exams
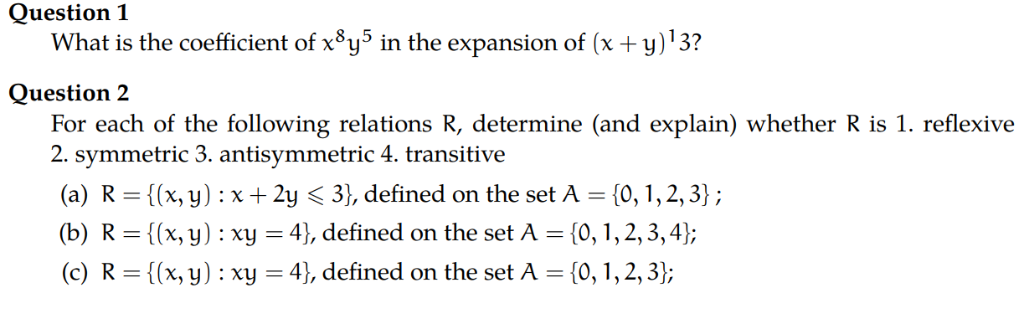



Question 1 What Is The Coefficient Of X8y5 In The Chegg Com
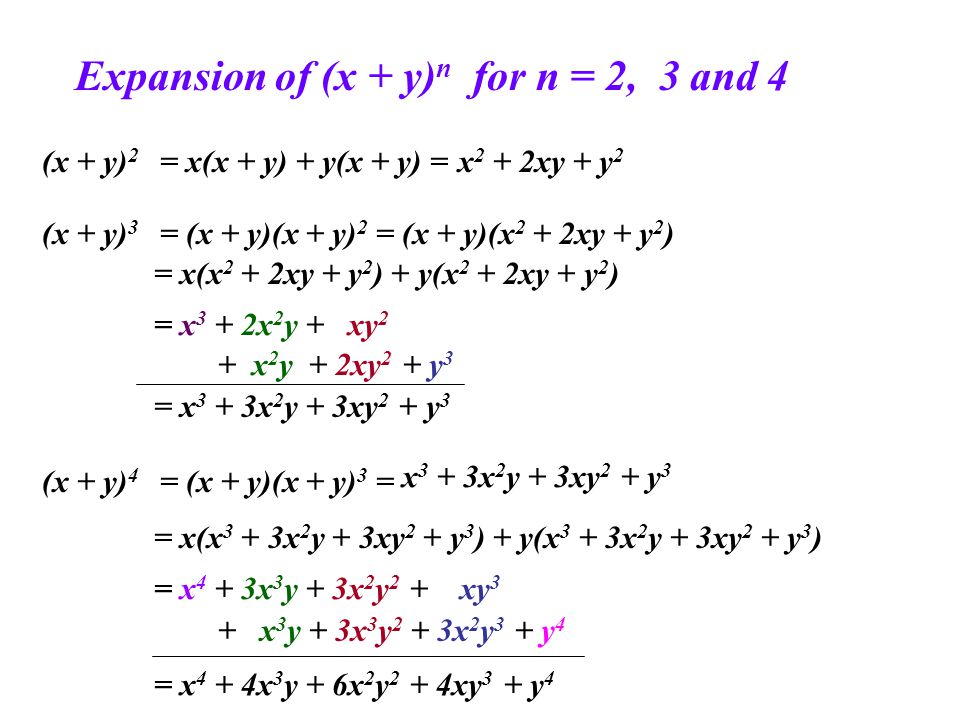



The Binomial Theorem 1 Objectives Pascal S Triangle Coefficient Of X Y N When N Is Large Notation Ncrncr Ppt Download
In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomialAccording to the theorem, it is possible to expand the polynomial (x y) n into a sum involving terms of the form ax b y c, where the exponents b and c are nonnegative integers with b c = n, and the coefficient a of each term is a specific positiveA commonly misunderstood topic in precalculus is the expansion of binomials In this video we take a look at what the terminology means, make sense of theQuestion Identify the binomial expansion of (xy)^3 Answer by rapaljer (4671) ( Show Source ) You can put this solution on YOUR website!



Myriorama Cards Were Invented In France Around 13
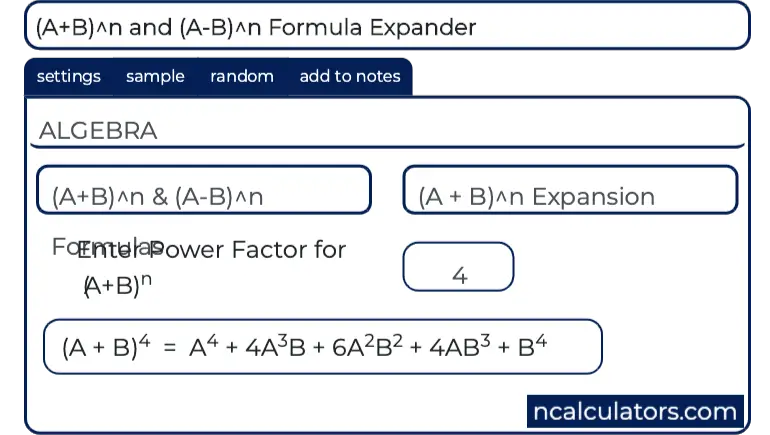



A B N And A B N Formula Expander



0 件のコメント:
コメントを投稿